[Uber][LeetCode] Interleaving String

Problem: Given strings s1, s2, and s3, find whether s3 is formed by an interleaving of s1 and s2.

An interleaving of two strings s and t is a configuration where s and t are divided into n and m substrings respectively, such that:

• s = s1 + s2 + ... + sn
• t = t1 + t2 + ... + tm
• | n - m | <= l
• The interleaving is s1 + t1 + s2 + t2 + s3 + t3 + ... or t1 + s1 + t2 + s2 + t3 + s3 + ...

Note: a + b is the concatenation of strings a and b.

Example:

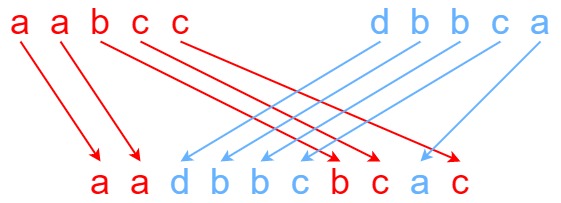
Input: s1 = "aabcc", s2 = "dbbca", s3 = "aadbbcbcac"
Output: true
Explanation: One way to obtain s3 is:
Split s1 into s1 = "aa" + "bc" + "c", and s2 into s2 = "dbbc" + "a".
Interleaving the two splits, we get "aa" + "dbbc" + "bc" + "a" + "c" = "aadbbcbcac".
Since s3 can be obtained by interleaving s1 and s2, we return true.

Input: s1 = "aabcc", s2 = "dbbca", s3 = "aadbbbaccc"
Output: false
Explanation: Notice how it is impossible to interleave s2 with any other string to obtain s3.

Input: s1 = "", s2 = "", s3 = ""
Output: true

Approach: We can simply try all the possible interleaving combinations of s1 and s2. We can use recursion to implement this approach. The algorithm will look like follows:

    

    IsInterleave(string s1, string s2, string s3, int s1Index, int s2Index, string currResult)

    {

        if (currResult == s3 && s1Index == s1.Length && s2Index == s2.Length)

        {

            return true;

        }

        

        bool result = false;

        

        if (s1Index < s1.Length)

        {

            result |= this.IsInterleave(s1, s2, s3, s1Index + 1, s2Index, currResult + s1[s1Index]);

        }

        

        if (s2Index < s2.Length)

        {

            result |= this.IsInterleave(s1, s2, s3, s1Index, s2Index + 1, currResult + s2[s2Index]);

        }

        

        return result;

    }

But the problem with the above problem is the time complexity. It will take 2^(m + n). Let's try to come up with better solution. If you see there are subproblems which we are solving multiple times. We can use DP here. The intuition of the solution is substring s3[0...k] can be interleaving of substring s1[0...i] and s2[0...j] does not depend on what comes after kth index in s3 or ith index of s1 and jth index of s2. 

We can maintain a 2D table where table[i][j] is true only if substring s3[0...i+j] is interleaving of s1[0...i] and s2[0...j] and we can use it for further calculation as s3[0...k] can't be interleaving of any substrings of s1 and s2 if s3[0...k-1] is not an interleaving.

Implementation in C#:

    public bool IsInterleave(string s1, string s2, string s3) 

    {

        if (s1.Length + s2.Length != s3.Length)

        {

            return false;

        }

        bool[,] table = new bool[s1.Length + 1, s2.Length + 1];

        for (int i = 0; i <= s1.Length; ++i)

        {

            for (int j = 0; j <= s2.Length; ++j)

            {

                if (i == 0 && j == 0)

                {

                    table[i, j] = true;

                }

                else if (i == 0)

                {

                    table[i, j] = table[i, j - 1] && s2[j - 1] == s3[i + j - 1]; 

                }

                else if (j == 0)

                {

                    table[i, j] = table[i - 1, j] && s1[i - 1] == s3[i + j - 1];

                }

                else

                {

                    table[i, j] = (table[i - 1, j] && s1[i - 1] == s3[i + j - 1]) || (table[i, j - 1] && s2[j - 1] == s3[i + j - 1]); 

                }

            }

        }

        return table[s1.Length, s2.Length];

    }

Complexity: O(m * n)